Mathematician Sir Michael Atiyah claims that he has proven the Riemann hypothesis concerning the distribution of Prime Numbers.

Sir Michael Atiyah is considered to be one of the World’s leading mathematicians; he has already received two of the highest awards in the field, the Fields Medal and the Abel Prize. And the Riemann hypothesis, that an equation known as the Riemann zeta function can produce all of the Prime Numbers, has been called math’s most important unsolved problem. So when a top mathematician announces that he has solved the biggest problem its major news. The image below is Sir Michael.

But that’s just what happened on the 24th of September on a stage at the Heidelberg Laureate Forum. At that forum Sir Michael presented what he himself described as a “radically new approach.” Dr. Atiyah’s proof will still have to be reviewed by other mathematicians before it will be accepted but the very possibility of that this problem could be solved has riveted the math world.

Now I don’t pretend to completely understand Sir Michael’s proof, yet. I just downloaded a copy and am working my way through it, remember I’m a physicist not a mathematician specializing in number theory. However I hope that I can explain something about prime numbers and why the Riemann hypothesis is so important.

We’ve all heard of prime numbers, those numbers that are divisible only by the number one 1 themselves. For example, while the number 6 can be written as 2×3, the number 7 can only be written as 1×7, 7 is a prime number. The prime numbers below 100 are given below. (one is traditionally not considered prime).

2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89 and 97.

Now take notice first of all that the number 2 is the only even prime number. That’s because all of the other even numbers can be divided by 2. You can also see how the distance between successive prime numbers is getting bigger, primes spread out as the numbers get bigger.

We’re not certain whether it was the Egyptians or Mesopotamians who first noticed that primes were different from other numbers but we do know that it was the Greeks, either Pythagoras or one of his students, who proved that there were an infinite number of primes. The proof is simple, and only takes three lines so what do you say. Shall we give it a try?

We will start by assuming that there is only a finite number of primes, let’s say p₁, p₂, p₃, through  p_n, that is we have n prime numbers total. Now we will demonstrate that that assumption is false. First we multiply all n of the prime numbers and add 1 to get, I’ll call it X.

X= (p₁ x p₂ x p₃ x  …x p_n) +1

Now, if we divide X by any of the primes, let’s say p_i there will always be a remainder of the 1/p_i. This means that either X must itself be prime, or evenly divided by some other prime not in our set of primes. In either case our assumption that we had all of the primes must be wrong, there are an infinite number of prime numbers.

O’k so how do we find those numbers that are prime? Is there some equation that will generate all of the primes for us?

Well, mathematicians searched for just such an equation for a very long time. Several partial equations, that is formulas that worked for a while, generating some primes were developed. One of the greatest mathematicians of all time, Bernhard Riemann worked for many years on prime number theory, his equation is known as the Riemann Zeta function. By the way Bernhard Riemann also developed the equations of geometry that Einstein used for his theory of gravity. The image below is Bernhard Riemann and beneath him is his Zeta function.

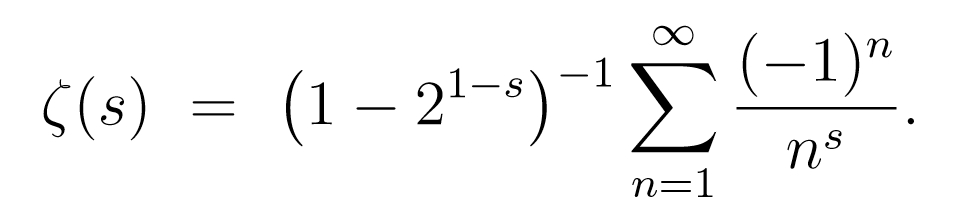
Riemann published his zeta function back in 1859 and in the years since then it has been checked, and confirmed, for the first 10,000,000,000,000 (that’s ten trillion!) primes but that doesn’t mean that it will work for all primes. Remember there are an infinite number of primes so ten trillion is actually not very many!

Sir Michael Atiyah claims to have proven that the zeta function works for all primes and if he’s right that will be a tremendous achievement. Other mathematicians now get to try to pull the proof apart. We’ll see what happens.